A story was on the BBC Wales news site recently where a motorist was filmed passing dangerously closely to a cyclist, tried to contest it and ended up losing and paying a massive fine.
One bit in the article that intrigued me was the motorist’s opinion was stated:
He said: “Taking into account the cyclist and his bike, there would have still been at least 4ft (1.2m) space.
https://www.bbc.co.uk/news/uk-wales-61815609
Which, ironically is admitting fault on his behalf (the minimum passing distance since 01 February is 1.5 m), but I was curious about how accurate he was.
Let’s see whether we find this out without travelling to the location.
Our first start is to play the video through and look for clues. This still has most of the information we need for our calculation:

The article thumbnail gives us a similar image without the overlaid text:

What do we know?
- From the still, the incident happened outside Heol-y-Cyw
- From the article, the car was an Audi Q8
- From the video and the shadow of the cyclist, the cyclist is in secondary position (near the verge) which normally varies from 0.30 to 0.50 m from the verge.
We can very quickly locate the location in Google maps, by searching for Heol-y-Cyw and confirming in Street View

The 40 mph decal on the road is really helpful here. From here we need to work out how much space was between the car and the cyclist, for this we need to work out the distance to the left side wheel. There’s a break in the tarmac of the road surface which the car’s left front tyre is straddling, roughly equally. We can’t see this from Google maps but we can from Street View. I’ve highlighted it in red.

We have several options for obtaining a standard “size”
- The size of speed limit roundel
- The width of the car
- The width of the carriageway
All three are valid techniques, which gives the advantage that we can use one technique and check with the others. I will cover each one below.
I’m going to make an assumption that as the cyclist is at secondary position, the point of the cyclist furthest from the verge is at 0.5 m. This is likely an underestimate, but there’s nothing firm in the video to show this.
Speed Limit Roundel
From the video or street view we can see a speed limit sign. Speed limit signs can vary a lot in size and still be legal, but usually when going from a national speed limit (i.e. 60 mph) to a 40 mph limit then we can refer to Traffic Signs Manual Chapter 3 (Regulatory Signs): https://assets.publishing.service.gov.uk/government/uploads/system/uploads/attachment_data/file/782724/traffic-signs-manual-chapter-03.pdf
On page 72 there’s a table to show the recommended sizes:
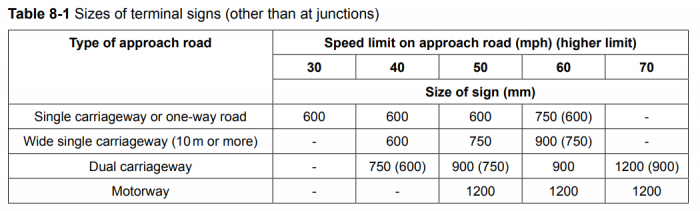
This means that the roundel is either 600 mm or 750 mm. The national speed limit sign on the other side is the same size, so I’m going to make the assumption that it is 600 mm, i.e. 0.60 m. This means that we can use this as a standard ruler, requiring more annotation.

The question here is how many green lines can I get into the purple line. We have to be careful. Because of the perspective from the mapping car there will be distortion – so there will be an error unless I can get the lines on the same horizontal line, which I probably haven’t.
Anyway, the green line goes from 1234 pixels to 1305 pixels, making it:
1305 – 1234 = 71 pixels = 0.6m
The purple line is 1053 to 881 pixels:
1053 – 881 = 172 pixels
And to convert this to metres we find the ratios between the pixels and multiply by the representation.
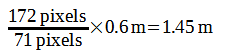
That’s already below the legal minimum, if we take the cyclist into account, then there was a gap of only:
1.45 m – 0.5 m= 0.95 m
Width of Car
I’ve added a purple line to the Street View image to approximate where the car is in the thumbnail. This was drawn by eye and may be slightly out. I’ve added a turquoise line to show the space between the car and the verge.

We know that the driver was driving an Audi Q8 which is (according to a number of sources, this is just one: https://www.autoexpress.co.uk/audi/q8/practicality) 1.995 m wide.
Assuming that my purple line is in the correct place we can measure the number of pixels and know that that equals 1.995 m. In this case my line ends at an x co-ordinate of 863 and starts at 676, therefore the line is:
863 – 676 = 187 pixels = 1.995 m
The next calculation is to measure the reminding space, which goes from 863 to 999 which is:
999 – 863 = 136 pixels
And as we know that 187 pixels is 1.995, we can work out the proportion and convert this to metres:
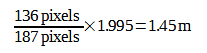
That matches the calculation above, so acts as a good confirmation that the maths is working out.
Width of Carriageway
This was the initial route I used to work out the distance, Google maps provides a scale which we can use to measure half the carriageway. Firstly, so make it easy, rotate the maps view so that section of the road is pointing upwards. Once again we annotate it.

From the scale, 20 m is 751 to 595 pixels, so:
751 – 595 = 156 pixels = 20 m
The red line goes from 375 to 350, so:
375 – 352 = 23 pixels
And converting to metres:
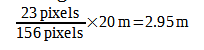
We can measure this on the streetview image, where the purple line is the width of the carriage way and the green is the space to the car:

You know what I’m going to do here, the purple line is:
1052 – 745 = 310 pixels = 2.95 m
The green line is:
1052 – 880 = 172 pixels
Converting to metres:
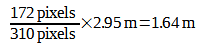
This is a bit different from the other two results, leading to a greater size, and a gap between the car and the cyclist which is still well under the legal minimum:
1.64 – 0.5 = 1.14 m
Why is this different? I’m taking more measurements, mostly taken by eye which could lead to a greater margin of error. If we make an assumption of a 10% margin of error to account for my dodgy eyesight that all figures are roughly equivalent.
Conclusion
So, what does this tell us? Not much really, some simple maths and a bit of knowledge can allow the use of a standard yardstick to make a guesstimate of a distance. There’s plenty missing in my calculations and a lot of assumptions and some missing bits, e.g. I didn’t even bother trying to compensate for the perspective in my Streetview images and a lot was performed manually.
Would I want to use this to present in a law court? No; there’s too much error using this technique, the only really safe would be to go to the site and measure. What if my assumptions about the cyclist’s position is wrong – there may be a wee bit more space.
Also there’s the lesson the driver needs to understand: he could have just moved over a bit more and overtaken the cyclist on the other side of the road and lost nothing. Treat cyclists like cars and give them space.